Index
- Introduction
- Integral Transforms of
- Solution of Generalized Fractional Kinetic Equation
- Alternate Solution of Generalized Fractional Kinetic Equation
- Special Cases
- Conclusion
- References
Abstract
Due to the great importance of the fractional kinetic equations, many authors discussed the generalizations of fractional kinetic equation involving various special functions. The purpose of this paper is to obtain the new generalization of fractional kinetic equation pertaining to the incomplete Aleph-function. The solution of the fractional kinetic equations obtained here by using Laplace and Sumudu transforms method. The Riemann-Liouville fractional integral operator is used to obtain the required results. The Solution of the generalized fractional kinetic equation are obtained by using the definition of incomplete Aleph function. The result discussed here can be used for the study of the chemical composition change in stars like the Sun. The solution rendered here are in compact forms suitable for numerical computation. Some special cases involving incomplete I –functions and incomplete H –functions are also considered.
Keywords: Fractional kinetic equations Incomplete Aleph- function Laplace transform Riemann-liouville fractional integral operator Sumudu transform.
Received: 6 December 2022 / Revised: 31 January 2023 / Accepted: 17 February 2023/ Published: 9 March 2023
Contribution/ Originality
In this paper author obtain the solution of fractional kinetic equation using incomplete Aleph function which is new and more significant in discussing some astrophysical problems. In order to obtain the solution of the fractional kinetic equations we have used the lemmas involving the Laplace and Sumudu Transforms and their inverse transforms.
1. INTRODUCTION
The fundamental equations of mathematical physics and natural science, the kinetic equations define the continuity of matter motion. Numerous significant problems in physics and astrophysics have been described and solved using fractional kinetic equations incorporating a large range of special functions. Numerous fractional operators were used in the extension and generalization of fractional kinetic equations [1-4] . The author presents a more generalized form of the fractional kinetic equation regarding incomplete Aleph-function in light of the effectiveness and significant role of the kinetic equation in some astrophysical situations.
Haubold and Mathai [1] created the fractional differential equation between the rate of change of the reaction, the destruction rate, and the production rate, which is stated as follows:
Haubold and Mathai [1] studied a special case of this equation, when instance fluctuation in quantity N(t) are neglected, is given by the equation:
The Sumudu transform of the Riemann-Liouville fractional integral of order v is defined as Belgacem, et al. [10]; Saxena, et al. [2]:
The Aleph function was first established in the 19th century by Sudland, et al. [11], and numerous fascinating findings and prospective applications in science, technology, and other disciplines were afterwards debated and extended by various authors.
A recent investigation and comprehensive research of the incomplete Pochhammer symbols was conducted by Srivastava, et al. [12]. They also discussed derivative formulae, Mellin-Barnes contour integral, generalized incomplete hypergeometric functions, and their integral representation. They also discussed potential applications in the areas of communication theory, groundwater pumping models, and probability theory.
The well-known incomplete gamma functions [13] are defined as:
Respectively, satisfy the subsequent decomposition formula:
Recently Kumar Bansal, et al. [14] introduce and investigate the incomplete Aleph –functions:
2. INTEGRAL TRANSFORMS OF 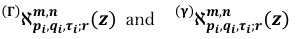
To solve generalized fractional kinetic equation, we use the Laplace transforms and Sumudu Transforms of incomplete Aleph –functions which are as follows:
Lemma 2.1. If
3. SOLUTION OF GENERALIZED FRACTIONAL KINETIC EQUATION
Theorem 3.1.
Is solvable and its solution is given by:
Proof. Proof is similar to the proof of theorem (3.1).
4. ALTERNATE SOLUTION OF GENERALIZED FRACTIONAL KINETIC EQUATION
In this part, we solve the generalized fractional kinetic equation by using Sumudu transform.
Theorem 4.1.
5. SPECIAL CASES
On setting ,the incomplete Aleph –function reduces to Aleph –function introduced by Südland and we obtain the results derived by Choi and Kumar [4].
If we take ,then (13) and (15) reduce to the incomplete I- functions introduced by Kumar Bansal, et al. [14] and we obtain the following results:
Corollary 5.1.
6. CONCLUSION
In this article, we have explored a new generalization of fractional kinetic equation. The solutions of fractional kinetic equation have been developed in terms of incomplete Aleph –function. Also, the particular cases hold the incomplete H-function, the incomplete I-function and familiar Aleph-function were discussed. The new generalized fractional kinetic equations presented here are general in nature and can be used in Statistical Mechanics and other branches of Mathematics.
Funding: This study received no specific financial support. |
Competing Interests: The author declares that there are no conflicts of interests regarding the publication of this paper. |
REFERENCES
[1] H. J. Haubold and A. M. Mathai, "The fractional kinetic equation and thermonuclear functions," Astrophysics and Space Science, vol. 273, no. 1, pp. 53-63, 2000.
[2] R. Saxena, A. Mathai, and H. Haubold, "On fractional kinetic equations," Astrophysics and Space Science, vol. 282, no. 1, pp. 281-287, 2002.
[3] V. Chaurasia and J. Singh, "Application of Sumudu transform in fractional kinetic equations, Gen," Mathematical Notes, vol. 2, no. 2, pp. 86-95, 2011.
[4] J. Choi and D. Kumar, "Solutions of generalized fractional kinetic equations involving Aleph functions," Mathematical Communications, vol. 20, no. 1, pp. 113-123, 2015.
[5] K. B. Oldhman and J. Spanier, The fractional calculus: Theory and applications of differentiation and integration to arbitrary order. New York: Academic Press, 1974.
[6] G. Samko, K. A. A., and O. I. Marichev, Fractional integrals and derivatives: Theory and applications. New York: Gordon and Breach Science Publishers, 1993.
[7] K. S. Miller and B. Ross, An introduction to the fractional calculus and fractional differential equations. New York: John Wiley and Sons, 1993.
[8] H. M. Srivastava and R. K. Saxena, "Operators of fractional integration and their applications," Applied Mathematics and Computation, vol. 118, no. 1, pp. 1-52, 2001. https://doi.org/10.1016/s0096-3003(99)00208-8
[9] G. K. Watugala, "Sumudu transform- a new integral transform to solve differential equations and control engineering problems," Mathematics Engineering Industrial, vol. 6, no. 4, pp. 319-329, 1998.
[10] F. B. M. Belgacem, A. A. Karaballi, and S. L. Kalla, "Analytical investigations of the Sumudu transform and applications to integral production equations," Mathematical Problems in Engineering, vol. 2003, no. 3, pp. 103-118, 2003. https://doi.org/10.1155/s1024123x03207018
[11] N. Sudland, B. Baumann, and T. F. Nonnenmacher, "Open problem: Who knows about the aleph (ℵ)-functions?," Fractional Calculus and Applied Analysis, vol. 1, pp. 401-402, 1988.
[12] H. Srivastava, M. A. Chaudhry, and R. P. Agarwal, "The incomplete Pochhammer symbols and their applications to hypergeometric and related functions," Integral Transforms and Special Functions, vol. 23, no. 9, pp. 659-683, 2012. https://doi.org/10.1080/10652469.2011.623350
[13] F. W. J. Olver, D. W. Lozier, R. F. Boisvert, and C. W. Clark, NIST digital library of mathematical functions; U.S. Department of commerce, national institute of standards and technology: Washington, DC, USA. Cambridge, UK: Cambridge University Press, 2010.
[14] M. Kumar Bansal, D. Kumar, K. Nisar, and J. Singh, "Certain fractional calculus and integral transform results of incomplete ℵ‐functions with applications," Mathematical Methods in the Applied Sciences, vol. 43, no. 8, pp. 5602-5614, 2020. https://doi.org/10.1002/mma.6299
[15] H. Srivastava, R. Saxena, and R. Parmar, "Some families of the incomplete H-functions and the incomplete $$\overline H $$ H¯-functions and associated integral transforms and operators of fractional calculus with applications," Russian Journal of Mathematical Physics, vol. 25, no. 1, pp. 116-138, 2018. https://doi.org/10.1134/s1061920818010119
Views and opinions expressed in this article are the views and opinions of the author(s), International Journal of Mathematical Research shall not be responsible or answerable for any loss, damage or liability etc. caused in relation to/arising out of the use of the content. |